Task Propensity

I’ve really enjoyed writing about task propensity over the last few months, and I’ve learned a ton about teaching and learning in the process. The series of posts was inspired by a paper on efforts to reform mathematics curriculum in the Netherlands. Here is the an excerpt from the introduction of the paper that got me started on this path:

In each case student performance did not match expectations, and in each case textbooks analysis revealed a focus on teaching effective procedures for specific tasks, instead of developing mathematical insights at a more advanced conceptual level as the reform intended. We will argue that this is at least in part due to what we call, “task propensity,” which we define as the tendency to think of instruction in terms of individual tasks that have to be mastered by students. This task propensity entices teachers and textbook authors to capitalize on procedures that can quickly generate correct answers, instead of investing in the underlying mathematics while accepting that fluency may come later.

The authors focus on reform curriculum — curriculum that was meant to support a more conceptual mathematical focus. I’m using task propensity more broadly; in the same way that teachers and curriculum writers fall into the trap of focusing on individual tasks rather than the underlying mathematics, students working on rich, engaging tasks fall into that same trap. In short, it is easy to lose the forest for the trees, and it’s hard to step back from individual problems to see the bigger picture.

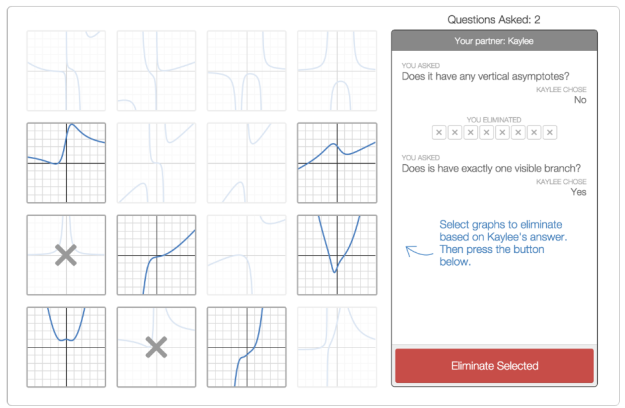
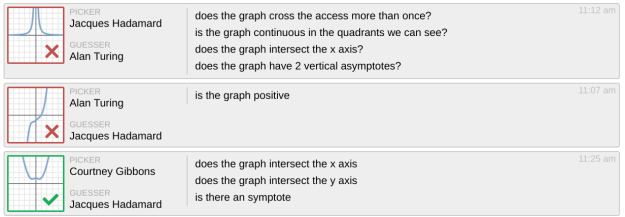
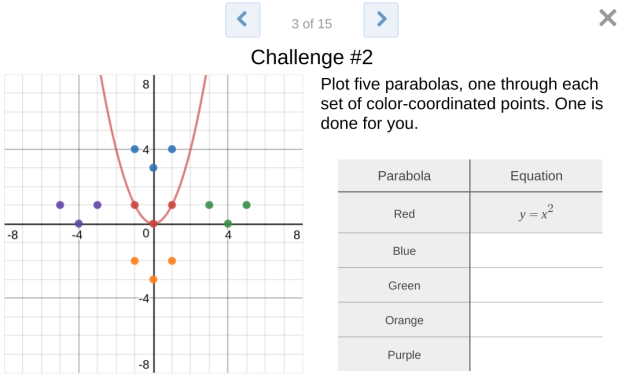
I was inspired to write this series because I see task propensity in my teaching all the time, both in the ways I structure tasks and activities and the ways students engage with them. I focused initially on Desmos activities because I think they can be particularly prone to task propensity, but I now see it in more and more places. I think task propensity is a natural human instinct, in which the practical concerns of solving the immediate problem supersede the learning that could be gained from stepping back, taking a broader perspective, and considering how the thinking in a task could be applied to new situations. I also think it’s inevitably a part of any ambitious and engaging curriculum.

I’ve explored three strategies for addressing task propensity that I think are also three useful design principles in any curriculum that focuses on students learning math by doing math — staying humble, follow-up tasks, and the pause.

Stay Humble 

When I stumble across a great lesson on the internet, it’s easy to focus on the allure of a fun activity that students will enjoy rather than the substance of the learning under the hood. That’s not to say student engagement is unimportant, just insufficient for meaningful learning. Staying humble means constantly calibrating my lens for what a great lesson is, focusing on substance over style. It means taking an ambitious lesson that is broad in scope and trimming it down to focus on a smaller number of well-defined mathematical goals. It means putting aside the big picture at times to zoom in on the building blocks that students need to support their larger understanding. Staying humble doesn’t mean teaching boring classes, but it does mean avoiding the temptation of sleek and sexy lessons when they’re just not the right tool for the job.

The Follow-Up Task 

Student engagement is great, but inevitably leads to a focus on the present rather than the future. In lots of tasks, that’s what I want, and I embrace the energy in the room. It also creates an opportunity for a deliberately designed follow-up task, where students return to a previous activity and consider its implications in a new problem. The initial task acts as an anchor to contextualize student thinking, whether they refer back to technology or manipulatives, borrow a bit of engagement from a fun experience, or reuse a useful problem type. Often during an engaging activity students are particularly engaged with the more “fun” elements of the task and not the underlying mathematics. Follow-up tasks take a step back from the incentives in the moment while returning to essential ideas that students can learn from.

Pause 

When students are caught up in an engaging activity I don’t instinctively want to stop them. I want to enjoy the moment and watch them have fun. At the same time, they’re also likely caught up in the activity in a way that prevents them from slowing down and thinking about how the math they’re doing might help them solve new problems in the future. While pausing them might elicit some groans, it also provides a great opportunity for students to think metacognitively about the connections they’re seeing and the math under the surface, rather than getting lost in the sauce of the moment-to-moment tasks. Pausing an activity is fundamentally about harnessing energy in the room to advance specific goals rather than leaving student thinking to chance.

Closing 

Spending this time exploring task propensity has helped me to think about teaching and learning in new ways. It’s an important reminder that kids learn what they spend time thinking about, and I want to plan my lessons deliberately to promote the type of thinking that will support new mathematical knowledge. Engagement is not the same as learning, but I can use student engagement and well-structured activities to create opportunities for students to do the thinking I want them to do. And student thinking should not be left up to chance — if I have a goal for students, I should modify or restructure the student experience to make sure they meet it.

Task Propensity: Beyond Desmos

Task propensity refers to situations where students are so focused on the features of a specific task that they don’t generalize their thinking in a way that is useful to solve different problems in the future. In short, they lose the forest for the trees. I’m exploring how task propensity relates to Desmos activities and how this thinking could help me teach more thoughtfully with Desmos tools. I first learned about task propensity through this paper, and you can read the rest of my series on the topic here.

---

 

When I first read about task propensity, I was interested because it described one of my hesitations with Desmos activities. The activities are engaging and fun for students, but that engagement didn’t always lead to the thinking that I wanted it to. I have spent some time this fall thinking about strategies to counteract that phenomenon — keeping activities humble, designing focused follow-up tasks, and pausing the activity.

At the same time as I’ve been practicing those strategies when I use Desmos activities, I’ve seen task propensity in other areas of my teaching. Any rich task can fall victim to a focus on the task itself rather than the broader mathematical thinking that goes into solving it. I want to explore two examples of task propensity in activities I’ve used, and how I might modify those activities the next time I teach them.

Trig War 

I want students to practice evaluating sine and cosine functions, but I don’t want that practice to be any more soul-deadening than it needs to be. Inspired by Kate Nowak’s Log War, I put together Trig War. Students pair up and divide the stack of cards in half. They then each flip over a card, figure out whose value is larger, and that person keeps both cards. Wash, rinse, repeat. It’s pretty fun and gets a lot of practice in. At the same time, some pairs end up relying on one person to evaluate tricky values, especially those outside the unit circle, or they end up rushing and missing opportunities to think about the structure of the unit circle and sine and cosine functions, focused more on the War than on the Trig.

One idea I want students to take away from Trig War is a stronger intuitive understanding of where things are on the unit circle. Students might look at two values and, without evaluating them, know by visualizing that one value is positive and the other is negative. They might recognize that cos(x) = cos(-x) based on the structure of the unit circle and use that property to quickly evaluate negative values for the cosine function. They might compare two values that are very close together on the unit circle without evaluating by thinking about whether that function is growing or shrinking on the relevant interval.

But in most cases, students don’t do this thinking. They’re too wrapped up in the game, and don’t want to slow down and look for mathematical structure. I can instigate the thinking I want using the same strategies I identified for task propensity. I can pause the game, have students put their cards down, and pose a sample comparison that elicits strategies I’m interested in. By slowing down and focusing on one question as a class, I avoid leaving student strategies to chance, and share with them some of the thinking I’d like to see from them. I can do something similar with a follow-up task — reinforcing a strategy we discussed as a group, and provoking extended reasoning and generalization in a way that is hard in a game context in the moment. And finally, I can try to keep the game as focused as possible. The version I have used in the past is ambitious — it addresses both sine and cosine functions, including negative values and positive values beyond the domain of the unit circle. I could consider splitting this up into two games — the first focused strictly on the unit circle, and the second expanding the domain to other values and stretching student thinking, so that each game can be laser focused on the specific strategies I want to elicit and the goals I set for students.

Sequences and Skittles 

I often begin a unit on sequences and series with a Skittles activity adapted from Julie Reulbach. Each group gets a package of Skittles, a plate, and a cup. They put a certain number of Skittles in the cup, shake them, place them on the plate, and then remove Skittles according to a rule — remove all Skittles with the “S” up, remove all Skittles with the “S” up then add five back, and more. Julie uses this activity to focus on decay and recursive functions, but I’ve adapted it to address other ideas as well.

It’s a ton of fun. There’s plenty of management involved to avoid making a complete mess, but it gets at neat examples of recursive functions that provoke some useful mathematical thinking. At the same time, that engagement can mean students are thinking more about Skittles and the excitement in the room than they are about the math.

The follow-up task becomes particularly important with this activity. Students can record data while they play with the Skittles, but asking them to do much more thinking while playing with the cup is likely to lead to rushed work and shallow reasoning. Instead, I see this activity as having two stages — the high-engagement initial task of playing with the Skittles and recording data, and then a follow-up task, once the Skittles are away, where students analyze and make connections with the specific math concepts I want to get at. This is also a great example of a place where I need to stay humble. I’ve tried to run this with multiple different versions of growth and decay with different recursive rules, but I think Julie was onto something by focusing on just two rules, both decaying. The more focused the investigation is, the more likely I am to get all students to reach my mathematical goals, and the less is left to chance. Finally, there’s the potential for some really great thinking while students are playing with the second rule Julie used — removing Skittles with the “S” up and adding five back. But just letting the activity run and stepping back leaves those a-ha moments to chance. Instead, I can time the activity more deliberately, get the majority of groups starting that experiment at the same time, and pause as they start to realize that the number of Skittles will likely never reach 0 to discuss with the whole class. These are subtle changes, but they’re changes that do a ton to focus student thinking on mathematical goals and minimize the task propensity of an engaging activity.

In Closing 

If there’s one lesson I want to take away from this, it’s that I need to constantly ask myself, “what are students thinking about right now?” Memory is the residue of thought. Task propensity happens when student thinking is focused too much on a specific task, and less on the mathematics behind that task. This was something I missed for a long time. I thought, “students are engaged, and there’s a lot of god thinking that could come out of this task”, and left it there. Now, I’m trying to think more about how to harness that engagement to make sure all students do exactly the thinking I’m interested in.

Task Propensity: Pause

Task propensity refers to situations where students are so focused on the features of a specific task that they don’t generalize their thinking in a way that is useful to solve different problems in the future. In short, they lose the forest for the trees. I’m exploring how task propensity relates to Desmos activities and how this thinking could help me teach more thoughtfully with Desmos tools. I first learned about task propensity through this paper, and you can read the rest of my series on the topic here.

I’ve been having a ton of fun with this series on task propensity. It’s fun because I love Desmos activities. I find them a joy to teach, I find them useful for student learning, and they let me do things that aren’t possible through other means. I also love thinking critically about where these activities are the right tools and where something else would work better. I probably spend about 15% of class time on Desmos activities, and that feels about right to me.

In my last two posts, I was critical of how Desmos activities fall into the trap of task propensity, and how keeping activities humble and designing deliberate follow-up tasks can help to avoid this challenge. In this post, I want to talk about the pause button — something that the folks at Desmos have baked into their interface and designed for student learning.

When the folks at Desmos talk about the pause button, they talk about classroom conversations and collective effervescence:

Collective effervescence is a term that calls to mind the bubbles in fizzy liquid. It’s a term from Émile Durkheim used to describe a particular force that knits social groups together. Collective effervescence explains why you still attend church even though the sermons are online, why you still attend sporting events even though they’re broadcast in much higher quality with much more comfortable seats from your living room. Collective effervescence explains why we still go to movie theaters; laughing, crying, or screaming in a room full of people is more satisfying than laughing, crying, or screaming alone.

There’s a ton of collective effervescence in Desmos activities. Take an activity I did recently, where students practice graphing sine and cosine functions by writing equations for different curves. There’s not much to it; it’s just meant to allow some low-floor practice early in the unit as we begin to formalize an understanding of how these functions work. Students like it a lot. It’s easy to experiment and change different parts of the function, and the challenges feel just within their reach.

Early in the activity, I noticed that most groups were using guess and check strategies to figure out the phase shifts. Take this screen:

One solution is to use a negative amplitude and then shift the function down. However, many groups end up trying to use a phase shift instead. This is a great opportunity for students to make connections through a quick discussion. Even better, some students write their phase shift in terms of pi, while others guess and check their way using decimals. Lots to draw on here.

Of course, at that moment there’s a ton of collective effervescence in the room. Students are engaged in the activity and in working collaboratively. It’s hard to tear them away. That’s where the pause button comes in.

There’s something really unique about the energy in the room when an activity is paused. There are often groans, and students wish they could keep working. It takes a moment to let that work its way out of their systems. The collective effervescence remains, but the task propensity — the feeling that students are “in it”, focused on meeting the challenges and nothing else — is put on pause as well. That excitement for math is great, but as students try to match the functions they see, they aren’t thinking about connections between different representations, the equivalence of multiple functions, or other big ideas of trigonometric functions I want them to learn. They’re stuck on the task in front of them.

The pause button is my chance to change their focus, to start a conversation with all the energy and engagement in the room that takes a step back, thinks more broadly about the math in front of students, and thinks about how some of the thinking that they’re doing could be applied in new contexts in the future.

When the pause button was added to the activity dashboard, I rarely used it. I felt like I didn’t want to interrupt student thinking and tear them away from what they wanted to do — from their goal of completing an activity they found enjoyable and challenging. I’ve shifted since then to see opportunities to redirect student thinking as the heart of my role as a teacher in that moment. My goal for a Desmos activity isn’t for students to get really good at Desmos. It’s for students to get really good at math. That means I need students to step out of the task, and to think metacognitively about what they’re doing and how it connects with what they already know and what they’ll do in the future. The pause button is by far the best tool I have for doing that.

Task Propensity: The Follow-Up Task

Task propensity refers to situations where students are so focused on the features of a specific task that they don’t generalize their thinking in a way that is useful to solve different problems in the future. In short, they lose the forest for the trees. I’m exploring how task propensity relates to Desmos activities and how this thinking could help me teach more thoughtfully with Desmos tools. I first learned about task propensity through this paper, and you can read the rest of my series on the topic here.

Polygraph

One example where I see task propensity degrade learning in engaging, well-intentioned activities is Polygraph. For context, Polygraph is a Guess Who-like game, where one student chooses a rational function among 16, and their partner asks yes or no questions to figure out which function they chose.

This is aided by technology to make the setup and transitions effortless and let me watch through the Desmos teacher dashboard. For instance, check out these student responses in Polygraph: Rational Functions:

We pause the activity and talk about vocabulary, looking at questions that worked well and making explicit the language that students could use to talk more precisely about the graphs they see. The activity is well-designed to create an intellectual need for vocabulary, and it’s effective for many students. At the same time, I still see responses like this through the end of the activity:

There are some missed opportunities here, and these types of exchanges persist for some students every time I run Polygraph.

The Follow-Up Task 

We finish with Polygraph, and the game and the computers go away. We maybe even wait a day for ideas to bounce around.  Then I give students this:

I don’t think this is anything brilliant or revolutionary. But I do think that spending 15 minutes working on this task in class and completing it for homework, perhaps over a few days, is worth far more than extending Polygraph for those additional minutes. The practice is more focused and less haphazard. I can have students trade with each other and try to find counterexamples, and suddenly there is even more need for precision in language, and more opportunity for students to revise their language and feel a need to add to their vocabulary for talking about rational functions. There’s even a more rigorous logic in writing questions with a much more challenging goal, and some classes students ask questions of the form, “say yes if the graph has two vertical asymptotes, say no if the graph has one vertical asymptote, and say I don’t know if the graph has no vertical asymptotes”, which I think is awesome. This task doesn’t work without Polygraph, but I think Polygraph is incomplete without some type of follow-up task to consolidate and extend student thinking.

Marbleslides

I love Marbleslides. Students love Marbleslides. They get to experiment with different functions, watch marbles roll around, and feel like they’re playing a game. Yet, despite the best intentions of Marbleslides’ design, students are guessing and checking far more than I would like. I love sequences of screens like these:

But too often students rush past these opportunities for reflection and consolidation of their learning to play with the marbles and the stars.

The Follow-Up Task 

Enter the follow-up task. I have students do Marbleslides, and a day or two later I give them this:

I get a lot of bang for my buck with this task. Students need to articulate differences between sine and cosine functions, and then write a function when they can’t see a full period of what they’re trying to model. Finally, they have to do all of that thinking without being able to guess and check their way to an answer. While this type of task is possible using the Pause and Teacher Pacing tools in Desmos activities, separating this task from the activity lets me take my time formatively assessing students strategies, selecting different approaches, sequencing them for a discussion, and unpacking different choices students made in writing their functions. And all of this happens without feeling like I’m interrupting students from playing a fun game in math class.

In short, I’ve found that Marbleslides is insufficient. It needs some bridges to connect work on Desmos with the thinking I need students to be able to do without the support of technology. There’s no substitute for Marbleslides in the scope and sequence of my curriculum. There’s also no substitute for a carefully designed follow-up task to reinforce and consolidate the big ideas, to make sure students don’t lose the forest for the trees.

More broadly, I find Desmos activities enormously valuable, but I also find them incomplete. They are incredibly engaging opportunities to work at formal and informal levels, experiment with a low floor, challenge students by raising the ceiling, and make connections through rich multimedia experiences. However, Desmos activities are only one element of a coherent curriculum, and there need to be bridges between those two — bridges that explicitly return to the thinking students do during a Desmos activity, consolidate it, and build on it toward larger goals.

 

Task Propensity: Stay Humble

Task propensity refers to situations where students are so focused on the features of a specific task that they don’t generalize their thinking in a way that is useful to solve different problems in the future. In short, they lose the forest for the trees. I’m exploring how task propensity relates to Desmos activities and how this thinking could help me teach more thoughtfully with those tools. I first learned about task propensity through this paper, and you can read the rest of my thinking on the topic here.

I think the best example of task propensity is Marbleslides.

Students solve challenges like the one above by rewriting the function so that when the balls drop, they capture all of the stars. I love Marbleslides and I use variations on it often. At the same time, I find that a subset of students — usually the students who are already struggling — learn less than I would hope through these tasks. They are likely to solve Marbleslides challenges through trial and error without paying attention to the structure of the mathematical objects they’re working with, or they get frustrated and use functions outside of the family I want them to learn about.

Marbleslides offers one paradigm for what Desmos activities can look like. These activities are incredibly engaging — students love them, and are often asking for more. They let students see math as a dynamic process, learning about objects that make sense and follow certain rules — and learning those rules is what learning math is all about. They are valuable activities and I’m glad I am able to use them.

But in this post I want to offer an alternate perspective that tries to avoid the challenge of task propensity. I spent a bunch of time this summer thinking about polynomials. My polynomial units often feel flat and uninspired and I wanted to add a wider variety of activities. I’ve previously used this Desmos activity.

Students solve challenges where they need to build functions that meet certain conditions. It can be great for certain features of polynomials, but can also suffer from some elements of task propensity. Students just end up fiddling with different functions until they find something that works, and in the process they may or may not learn what I want them to learn about polynomials.

I wanted to design a new activity, one that falls after Polygraph at the start of the unit but before students start doing more formal algebraic work. My goal was to bridge some of the gaps between using vocabulary to describe polynomial functions and writing polynomial functions that meet certain conditions. I also wanted to write something humble. There’s nothing very flashy about this activity, no high-engagement tasks that students will want to keep coming back to. I want this activity to provoke useful thinking, and to do so using tools like sketching and interactive Desmos graphs that are impossible with a pencil and paper or whiteboard and marker. And I want it to stay laser focused on a few key ideas that I want to get across. I’m having trouble clearly articulating what I like about this activity that I don’t find in some others, but I’ll try to lay out what I was going for below. The activity is linked here if you’d like to play along.

Below are the first two screens. They are meant to give me a rough idea of how my students conceptualize polynomials.

I would use teacher pacing here, so that students can only work on these two screens and can’t go further ahead. Then, I would pause and project a few examples anonymously to discuss why they are or are not polynomials, and show students a few different ideas of what the function could look like. Nothing crazy, just trying to see where student thinking is and help them do some informal work sketching and seeing sketches of polynomials.

The next four screens are also formative, and are more focused on multiplicity, where I find many students get tripped up when working with polynomials. I would use teacher pacing on these screens as well so that students can’t go ahead.

The goal here is to explore how even and odd multiplicity influence a function, and see how well students can sketch a function that has specific characteristics while connecting multiplicity to other properties of that function. Nothing too crazy, but also something that I can only let students experiment with informally through a Desmos activity. They’re also meant to be really carefully focused on an informal understanding of multiplicity, making sure students are doing the right thinking on these screens. There’s a great opportunity to share different students’ graphs with the whole group and discuss both the specific properties and how they come together to create the larger function.

Next they would likely finish the activity at their own pace. I don’t want work through the potential management challenges of continuing teacher pacing. I’m watching the dashboard and looking for two things: interesting disagreements or misconceptions to surface and discuss at the end of the activity, and where student thinking is more broadly as I figure out what to do after this activity.

I think this is far from perfect and some of its rough edges could be smoothed out. But this lesson sticks with some values I want to try to use more often with Desmos activities. It isn’t trying to tour through an entire concept in 45 minutes. It isn’t supposed to be my most engaging lesson. Instead, the goal is to be laser focused on an important development in student thinking — reasoning flexibly about polynomial graphs and the vocabulary we use to describe them, without getting into algebraic notation. By staying really focused and living in that specific place, I’m trying to avoid some of the challenges of task propensity. There are no fancy challenges that students have to work through. The focus is on sketching and explaining their thinking. There is less emphasis on guess-and-check than many other Desmos activities. And I built this activity thinking specifically about how I want to use teacher pacing and other Desmos conversation tools in order to create useful moments of formative assessment and class discussion.

I don’t mean this to be a criticism of other Desmos activities, just a change in emphasis for me on what is missing in some of my pedagogy. It’s also meant to be something that complements what I’m already doing, rather than replacing other activities. There’s a time for engagement and excitement, and there’s a time for humble activities that zoom in on specific goals and focus on getting all students to meet those goals.

I would love feedback. Is this a distinction worth making? Is this activity really just a mess? Where else might this type of thinking be useful? Where could I go further?

Task Propensity & Rich Tasks

I wrote a few days ago about Desmos and task propensity. I’m interested in critically exploring the pedagogy and sequencing behind using Desmos activities effectively.  One challenge is the idea of task propensity: when presented with a conceptually-oriented task, teachers and students often focus too much on the thinking that will help them solve the specific task, rather than thinking that will help them connect what they are doing to new problems in the future.  If students are laser-focused on finding the solution to a problem, they are likely to lose the forest for the trees and miss opportunities to generalize their thinking.

Before I dive deeper into this idea, I want to explore the opposite perspective. Humans inevitably focus on the task at hand and push to the background thinking about how they will use it again in the future. This isn’t a problem unique to Desmos activities. And, if it’s in some ways inevitable, we might as well make the tasks that students are focusing on as rich, engaging, and meaningful as possible.

We talked a bit during the Desmos fellows weekend about ways that technology can help and hinder learning. There are lots of ways it can hinder learning — by isolating students, by presenting new distractions, etc. There are also lots of ways it can help learning — it can give immediate feedback, differentiate by providing multiple entry points and tiered challenges, and etc. But the heart of what I find most valuable in this technology is that I can give students richer tasks that create richer thinking with technology than without.

The phrase “rich task” is often thrown around but under-specified. I’m not sure I have a great definition, but I’d like to offer a few examples of what can make a Desmos activity a rich task.

Match My Parabola 

Here is a screen from the Match My Parabola activity:

I’m not sure I can even offer an alternative that is equivalent. Here, students can experiment and instantly see what graphs their equations produce. At the same time, once they figure out how to transform the quadratic function vertically or horizontally, they practice that transformation once more in a slightly different location. That sequencing — experimentation until students find success, and then immediate practice — is pretty hard to replicate elsewhere. Desmos does it smoothly and seamlessly.

Game, Set, Flat 

Here is a screen from the Game, Set, Flat activity:

Students have been introduced to the challenge: they need to be able to tell good tennis balls from bad tennis balls. They just saw a few examples of balls bouncing, with varying levels of bounciness, to help illustrate the difference. Before students work formally with equations, they have a chance to get a firmer grasp on the principles involved by choosing how high a ball bounces after each bounce. When they click the button, they see their model animated. It animates whether they create a close approximation of a bouncing ball or something silly and unreasonable. But this intermediate step helps students to visualize the problem and sets them up to make connections between equations and the objects they represent. Most importantly, this is something that is impossible to do without digital technology; this whole step is skipped in a pencil-and-paper lesson, and students miss out on the chance to do this thinking.

Burning Daylight 

Here are two consecutive screens in the activity Burning Daylight:


It’s easy to stay focused on the abstractions and equations when engaging with mathematical modeling. The first question would, in many paper-and-pencil lessons, be one that students rush past on the way to writing a quick equation and moving on. In this case, the media allows immediate feedback contextualizing the student’s thinking on the previous screen and reinforcing a connection between model and world that might otherwise be lost.

Summary 

These are great tasks. And they’re great tasks because they have the potential to create rich thinking for students, in ways that aren’t possible in other formats.

One challenge that I’m interested in exploring is this idea of task propensity: to what extent do students, while working on these activities, focus on the tasks themselves without stepping back to consider how they can use what they’ve learned in new contexts in the future? That said, even if students have a hard time slowing down to make connections I would like them to make, the thinking that they need do to complete these tasks is richer, deeper, and more varied than the best replacements I could offer.

I want to offer this mostly as a check for myself. If I want to take a critical perspective on Desmos activities, I want to make sure I’m clear on exactly what they offer, what they are being compared to, and what my alternatives are.

Task Propensity

This task propensity entices teachers and textbook authors to capitalize on procedures that can quickly generate correct answers, instead of investing in the underlying mathematics while accepting that fluency may come later.

(source)

The article linked above is a thought-provoking perspective on why some conceptually-focused math reforms have been unsuccessful. The authors explore the idea of task propensity, or the tendency of teachers and curriculum writers to focus on features of specific tasks rather than  the underlying mathematics that may be used in new tasks in the future. Teachers may have great, conceptually oriented tasks that can elicit mathematical thinking, yet if they only focus on teaching students how to solve those specific tasks that thinking is unlikely to transfer to new problems down the road.

I’m hanging out with some great folks at the Desmos fellows weekend, and I’d like to share two contrasting cases:

Case 1 
We spent some time yesterday mingling and doing math together. I spent much if working on this problem from Play With Your Math with a great group of teachers.

I won’t spoil it; this is absolutely worth exploring, and after what was probably an hour of work I have plenty more to learn. The most important feature of my learning was that, in a relatively short period of time, the group I was working with established the answer to the question as it was posed. We then went further, and explored different conjectures and directions to extend the problem. The vast majority of our learning came after we had solved the problem, and depended on our interest in creating new problems to further our thinking. In other words, we avoided the temptation of task propensity to fixate on the problem at the expense of additional learning.

Case 2:
I have really enjoyed both playing and watching students play Marbleslides lessons like this one. Students have to transform various functions in order for the marbles to get every star when they are launched.

This is one of my students’ favorite things to do in class, and is far more engaging for them than any other lesson I have on rational functions. At the same time, I find that students often learn less than I would like from the activity. They spend most of their time focused on the task at hand — getting all of the stars — and less on what I want them to learn — general rules for transforming rational functions. This is not to say that no learning happens, just that students can fall victim to task propensity and lose the forest for the trees.

I am looking forward to my Desmos fellowship and what I will learn from a great group of teachers and the stellar folks at Desmos. One of the important questions I have is around when Desmos is the appropriate tool to use, and when other tools will work just as well or better. One challenge I have with many activities is task propensity; that, while Desmos is a powerful tool for generalizing thinking, that generalization does not happen if students are too focused on the specific features of a task to make connections to broader mathematical ideas. I hope to do some writing over the next few months to explore this idea and try to better understand when Desmos is the right tool, and how to use it effectively.